1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134
135
136
137
138
139
140
141
142
143
144
145
146
147
148
149
150
151
152
153
154
155
156
157
158
159
160
161
162
163
164
165
166
167
168
169
170
171
172
173
174
175
176
177
178
179
180
181
182
183
184
185
186
187
188
189
190
191
192
193
194
195
196
| # AW 2014-03-17
# GPLv2+ license
import math
import matplotlib.pyplot as plt
import numpy
# first order trajectory. bounded velocity.
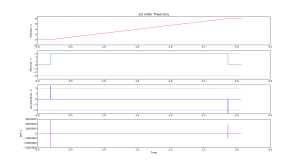
class Trajectory1:
def __init__(self, ts = 1.0, vmax = 1.2345):
self.ts = ts # sampling time
self.vmax = vmax # max velocity
self.x = float(0) # position
self.target = 0
self.v = 0 # velocity
self.t = 0 # time
self.v_suggest = 0
def setTarget(self, T):
self.target = T
def setX(self, x):
self.x = x
def run(self):
self.t = self.t + self.ts # advance time
sig = numpy.sign( self.target - self.x ) # direction of move
if sig > 0:
if self.x + self.ts*self.vmax > self.target:
# done with move
self.x = self.target
self.v = 0
return False
else:
# move with max speed towards target
self.v = self.vmax
self.x = self.x + self.ts*self.v
return True
else:
# negative direction move
if self.x - self.ts*self.vmax < self.target:
# done with move
self.x = self.target
self.v = 0
return False
else:
# move with max speed towards target
self.v = -self.vmax
self.x = self.x + self.ts*self.v
return True
def zeropad(self):
self.t = self.t + self.ts
def prnt(self):
print "%.3f\t%.3f\t%.3f\t%.3f" % (self.t, self.x, self.v )
def __str__(self):
return "1st order Trajectory."
# second order trajectory. bounded velocity and acceleration.
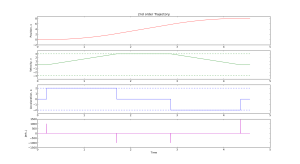
class Trajectory2:
def __init__(self, ts = 1.0, vmax = 1.2345 ,amax = 3.4566):
self.ts = ts
self.vmax = vmax
self.amax = amax
self.x = float(0)
self.target = 0
self.v = 0
self.a = 0
self.t = 0
self.vn = 0 # next velocity
def setTarget(self, T):
self.target = T
def setX(self, x):
self.x = x
def run(self):
self.t = self.t + self.ts
sig = numpy.sign( self.target - self.x ) # direction of move
tm = 0.5*self.ts + math.sqrt( pow(self.ts,2)/4 - (self.ts*sig*self.v-2*sig*(self.target-self.x)) / self.amax )
if tm >= self.ts:
self.vn = sig*self.amax*(tm - self.ts)
# constrain velocity
if abs(self.vn) > self.vmax:
self.vn = sig*self.vmax
else:
# done (almost!) with move
self.a = float(0.0-sig*self.v)/float(self.ts)
if not (abs(self.a) <= self.amax):
# cannot decelerate directly to zero. this branch required due to rounding-error (?)
self.a = numpy.sign(self.a)*self.amax
self.vn = self.v + self.a*self.ts
self.x = self.x + (self.vn+self.v)*0.5*self.ts
self.v = self.vn
assert( abs(self.a) <= self.amax )
assert( abs(self.v) <= self.vmax )
return True
else:
# end of move
assert( abs(self.a) <= self.amax )
self.v = self.vn
self.x = self.target
return False
# constrain acceleration
self.a = (self.vn-self.v)/self.ts
if abs(self.a) > self.amax:
self.a = numpy.sign(self.a)*self.amax
self.vn = self.v + self.a*self.ts
# update position
#if sig > 0:
self.x = self.x + (self.vn+self.v)*0.5*self.ts
self.v = self.vn
assert( abs(self.v) <= self.vmax )
#else:
# self.x = self.x + (-vn+self.v)*0.5*self.ts
# self.v = -vn
return True
def zeropad(self):
self.t = self.t + self.ts
def prnt(self):
print "%.3f\t%.3f\t%.3f\t%.3f" % (self.t, self.x, self.v, self.a )
def __str__(self):
return "2nd order Trajectory."
vmax = 3 # max velocity
amax = 2 # max acceleration
ts = 0.001 # sampling time
# uncomment one of these:
#traj = Trajectory1( ts, vmax )
traj = Trajectory2( ts, vmax, amax )
traj.setX(0) # current position
traj.setTarget(8) # target position
# resulting (time, position) trajectory stored here:
t=[]
x=[]
# add zero motion at start and end, just for nicer plots
Nzeropad = 200
for n in range(Nzeropad):
traj.zeropad()
t.append( traj.t )
x.append( traj.x )
# generate the trajectory
while traj.run():
t.append( traj.t )
x.append( traj.x )
t.append( traj.t )
x.append( traj.x )
for n in range(Nzeropad):
traj.zeropad()
t.append( traj.t )
x.append( traj.x )
# plot position, velocity, acceleration, jerk
plt.figure()
plt.subplot(4,1,1)
plt.title( traj )
plt.plot( t , x , 'r')
plt.ylabel('Position, x')
plt.ylim((-2,1.1*traj.target))
plt.subplot(4,1,2)
plt.plot( t[:-1] , [d/ts for d in numpy.diff(x)] , 'g')
plt.plot( t , len(t)*[vmax] , 'g--')
plt.plot( t , len(t)*[-vmax] , 'g--')
plt.ylabel('Velocity, v')
plt.ylim((-1.3*vmax,1.3*vmax))
plt.subplot(4,1,3)
plt.plot( t[:-2] , [d/pow(ts,2) for d in numpy.diff( numpy.diff(x) ) ] , 'b')
plt.plot( t , len(t)*[amax] , 'b--')
plt.plot( t , len(t)*[-amax] , 'b--')
plt.ylabel('Acceleration, a')
plt.ylim((-1.3*amax,1.3*amax))
plt.subplot(4,1,4)
plt.plot( t[:-3] , [d/pow(ts,3) for d in numpy.diff( numpy.diff( numpy.diff(x) )) ] , 'm')
plt.ylabel('Jerk, j')
plt.xlabel('Time')
plt.show() |