Here's an experiment I've done recently:
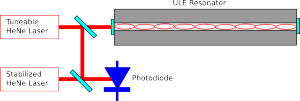
(Time-lapse of ca 18 hour experiment. Bottom left is a spectrum-analyzer view of the beat-note signal. Top left is a frequency counter reading of the beat-note. Bottom right is a screen showing the a camera-view of the output-beam from the resonator)
This is a measurement of the thermal expansion of a fancy optical resonator made from Corning "Ultra Low Expansion" (ULE) glass. This material has a specified thermal expansion of 0.03 ppm/K around room temperature. This thermal expansion is roughly 800-times smaller than Aluminium, around 400-times smaller than Steel, and 40-times better than Invar - a steel grade specifically designed for low thermal expansion.
Can we do even better? Yes! Because ULE glass has a coefficient of thermal expansion (CTE) that crosses zero. Below a certain temperature it shrinks when heated, and above the zero-crossing temperature it expands when heated (like most materials do). This kind of behavior sounds exotic, but is found is something as common as water! (water is heaviest at around 4 C). If we can use the ULE resonator at or very close to this magic zero-crossing temperature it will be very very insensitive to small temperature fluctuations.
So in the experiment I am changing the temperature of the ULE glass and looking for the temperature where the CTE crosses zero (let's call this temperature T_ZCTE). The effect is fairly small: if we are 1 degree C off from T_ZCTE we would expect the 300 mm long piece of ULE glass to be 200 pm (picometers) longer than at T_ZCTE. That's about the size of a single water-molecule, so this length change isn't exactly something you can go and measure with your digital calipers!
Here's how it's done (this drawing is simplified, but shows the essential parts of the experiment):
We take a tuneable HeNe laser and lock the frequency of the laser to the ULE-cavity. The optical cavity/resonator is formed between mirrors that are bonded to the ends of the piece of ULE glass. We can lock the laser to one of the modes of the cavity, corresponding to a situation where (twice) the length of the cavity is an integer number of wavelenghts. Now as we change the temperature of the ULE-glass the laser will stay locked, and as the glass shrinks/expands the wavelength (or frequency/color) of the laser will change slightly.
Directly measuring the frequency of laser light isn't possible. Instead we take second HeNe laser, which is stabilized to have a fixed frequency, and detect a beat-note between the stabilized laser and the tuneable laser. The beat-note will have a frequency corresponding to the (absolute value of the) difference in frequency between the two lasers. Now measuring a length-change corresponding to the size of a single water-molecule (200 pm) shouldn't be that hard anymore!
Let's say the stabilized laser has a wavelength of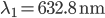 
(red light). Its frequency will be 
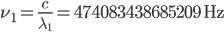(that's around 474 THz). When the tuneable laser is locked to the cavity we force its wavelength to agree with 
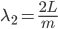where 
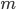is an integer and 
is the length of the cavity. I've drawn only a small number of wavelengths in the figure, but a realistic integer is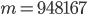 
. We get 
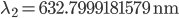and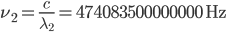 
, very nearly but not quite the same wavelength/frequency as the stabilized laser. Now our photodiode which measures the beat-note will measure a frequency of 
.
How does this change when the ULE glass expands by 200 pm? When we heat or cool the cavity by 1 degree C the length changes to 300 mm + 200 pm, and the wavelength of the tuneable laser will change to
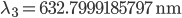. Now our beat-note detector will show 
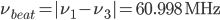. That's a change in the beat-note of more than 300 kHz - easily measurable!
That's how you measure a length-change corresponding to the diameter of a water molecule!
Why do this? Some of the best ultra-stable lasers known are made by locking the laser to this kind of ULE-resonator. Narrow linewidth ultra-stable lasers are interesting for a host of atomic physics and other fundamental physics experiments.
See also: Janis Alnis playing with two ultra-stable lasers.
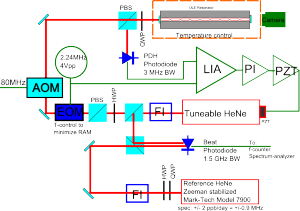
Update 2013 August: I made a drawing in inkscape of the experimental setup.
This figure shows most (if not all?) of the important components of this experiment. The AOM is not strictly required but I found it useful to shift the tuneable HeNe laser by +80 MHz to reach a TEM-00 mode of the ULE resonator. Not shown is a resonance-circuit (LC-tank) between the 2.24MHz sinewave-generator and the EOM. The EOM was temperature controlled by a TEC with an NTC thermistor giving temperature feedback.
Hi Anders,
thats very interesting experiment though...as I thought of similar, needed to proof (or dis-proof) an idea I have regarding gravity.
It is related to a VLS Theory (one of many already) , so a varying lightspeed theory. However, always the issue was the stability of Lasers like HeNes in regard to temperature changes. Ideal would be, to have two HeNe's made of this ULE glass. Do you know if such existing? Otherwise I could redesign the experiment in a way to use such cavities as you did in your experiment. I would rather ruse yet lower beat notes, to have higher resolution in measurement, as the effect is pretty small: 10^-8 of the Oscillators frequency.
The experiment would aim at showing that lightspeed is over a height difference not constant and gravitation just and only based on this change in speed of light.
Thanks for your attention and hope to hear from you.
Heinrich
Hi Heinrich,
This kind of ULE cavity is quite commonly used for optical clock experiments. Recent high-end experiments also use single-crystal Si for the cavity, but the zero thermal expansion temperature is cryogenic (either 124K or 4K IIRC) - so makes things technically more complicated.
As far as I know there is no advantage to making the laser-cavity itself from ULE, the standard approach is to use e.g. an ECDL laser and lock it to an external cavity. The wavelenghts are typically chosen where very good mirrors are available - it is usually good to have as high mirror reflectance, and thus cavity Q/finesse, as possible.
ULE cavities typically shrink throughout their lifetime, even if held at a temperature where the thermal expansion is zero. We get values of ca -10 mHz/s isothermal drift for our laser at 674nm. These things are very sensitive to vibrations, sound, etc. - so if you plan an experiment where you move one cavity up/down a lot then it will be challenging. There's a number of papers on clock-lasers for transportable optical clocks (even in space) which may give you some clues.